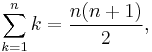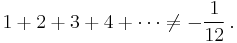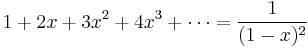1 + 2 + 3 + 4 + …
The sum of all natural numbers 1 + 2 + 3 + 4 + · · · is a divergent series. The nth partial sum of the series is the triangular number
which increases without bound as n goes to infinity.
Although the full series may seem at first sight not to have any meaningful value, it can be manipulated to yield a number of mathematically interesting results, some of which have applications in other fields such as complex analysis, quantum field theory and string theory. For example, zeta function regularization gives the result
Furthermore, the sum is always an even perfect number if and only if n = 2p-1 is a Mersenne prime, in which case p is a prime number.
Contents |
Summability
Unlike its alternating counterpart 1 − 2 + 3 − 4 + · · ·, the series 1 + 2 + 3 + 4 + · · · is not Abel summable. Its generating function
has a pole at x = 1.
The series can be summed by zeta function regularization. When the real part of s is greater than 1, the Riemann zeta function of s equals the sum 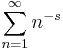 . This sum diverges when the real part of s is less than or equal to 1, but when s = −1 then the analytic continuation of ζ(s) gives ζ(−1) as −1/12.
. This sum diverges when the real part of s is less than or equal to 1, but when s = −1 then the analytic continuation of ζ(s) gives ζ(−1) as −1/12.
The Ramanujan sum of 1 + 2 + 3 + 4 + · · · is also −1/12.[1] In Srinivasa Ramanujan's second letter to G. H. Hardy, dated 27 February 1913, he wrote:
- "Dear Sir, I am very much gratified on perusing your letter of the 8th February 1913. I was expecting a reply from you similar to the one which a Mathematics Professor at London wrote asking me to study carefully Bromwich's Infinite Series and not fall into the pitfalls of divergent series. … I told him that the sum of an infinite number of terms of the series: 1 + 2 + 3 + 4 + · · · = −1/12 under my theory. If I tell you this you will at once point out to me the lunatic asylum as my goal. I dilate on this simply to convince you that you will not be able to follow my methods of proof if I indicate the lines on which I proceed in a single letter. …"[2]
Physics
In bosonic string theory, the attempt is to compute the possible energy levels of a string, in particularly the lowest energy level. Speaking informally, each harmonic of the string can be viewed as a collection of 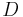 independent quantum harmonic oscillators, where
independent quantum harmonic oscillators, where 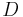 is the dimension of spacetime. If the fundamental oscillation frequency is
is the dimension of spacetime. If the fundamental oscillation frequency is 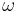 then the energy in an oscillator contributing to the
then the energy in an oscillator contributing to the  th harmonic is
th harmonic is 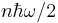 . So using the divergent series, the sum over all harmonics is
. So using the divergent series, the sum over all harmonics is 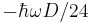 . Ultimately it is this fact, combined with the no-ghost theorem, which leads to bosonic string theory failing to be consistent in dimensions other than 26.
. Ultimately it is this fact, combined with the no-ghost theorem, which leads to bosonic string theory failing to be consistent in dimensions other than 26.
A similar calculation is involved in computing the Casimir force.
See also
- Triangular number
- Infinite arithmetic series
- 1 + 1 + 1 + 1 + · · ·
- 1 − 2 + 3 − 4 + · · ·
- 1 + 2 + 4 + 8 + · · ·
Notes
References
- Berndt, Bruce C., Srinivasa Ramanujan Aiyangar, and Robert A. Rankin (1995). Ramanujan: letters and commentary. American Mathematical Society. ISBN 0-8218-0287-9.
- Hardy, G.H. (1949). Divergent Series. Clarendon Press. LCC QA295 .H29 1967.
Further reading
- Lepowsky, James (1999). "Vertex operator algebras and the zeta function". Contemporary Mathematics 248: 327–340. arXiv:math/9909178.
- Zee, A. (2003). Quantum field theory in a nutshell. Princeton UP. ISBN 0-691-01019-6. See pp. 65–6 on the Casimir effect.
- Zwiebach, Barton (2004). A First Course in String Theory. Cambridge UP. ISBN 0-521-83143-1. See p. 293.